You may be familiar with converting between different units (e.g. m and cm, or m and km), but how do we apply this when we are also calculating areas?
When we are converting from, for example, metres into cm, we know that 1 m = 100 cm.
This can lead to the misconception that 1 m2 = 100 cm2.
BUT, in reality, 1 m2 = 10,000 cm2 as we need to take into account our area conversion factors.
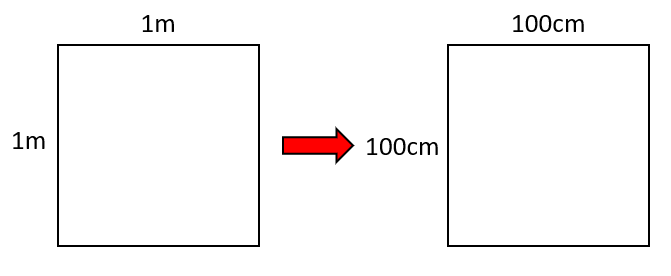
Let's look at what we mean here with a diagram to help.
If we look at a square that measures 1 m on each side:
Looking at the areas of these two identical squares, we can say that:
1 m × 1 m = 100 cm × 100 cm
1 m2 = 10,000 cm2
Is there a trick?
In a way, yes.
All we need to do for an area conversion is to find the conversion if it was a length, and then square it to get the area conversion.
Let's look at an example to see this in action.
e.g. Convert 1.4 m2 into cm2
We know that for length, 1 m = 100 cm
So for area, 1 m2 = 10,000 cm2
So 1.4 m2 = 1.4 × 10,000 = 14,000 cm2
e.g. Convert 350 m2 into km2
We know that for a length, 1 km = 1000 m
So for area, 1 km2 = 1,000,000 m2
So 350 m2 = 350 ÷ 1,000,000 = 0.00035 m2
In this activity, we will convert areas (expressed in cm2, m2 and km2) into alternative units of measurement by applying the area conversion factor using the methods shown above.