Identify Right-Angled Triangles

Pythagoras' theorem states that in any right-angled triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides.

 

 

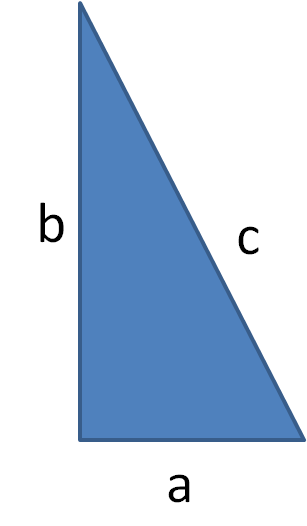
Thus in the above right-angled triangle a² + b²  = c² 

 

If a triangle of sides a, b and c is such that a² + b² = c², then the triangle is right-angled.

 

Example 1

A triangle has sides of length 6 cm, 8 cm and 9 cm.

Is it right-angled?

 

Answer

First, pick the two shorter sides, square them and then add:

6² + 8² = 36 + 64 = 100

 

Then square the longest side:

9² = 81

So 6² + 8² ≠ 9² and the triangle is therefore not right-angled.

 

Example 2

A triangle has sides of length 5 cm, 13 cm and 12 cm.

Is it right-angled?

 

Answer

First, pick the two shorter sides, square them and then add:

5² + 12² = 25 + 144 = 169

 

Then square the longest side:

13² = 169

 

So 5² + 12² = 13² and the triangle is therefore right-angled.

 

Don't worry too much if you feel unsure or overloaded with information.

We will work through ten questions together so that you feel super confident